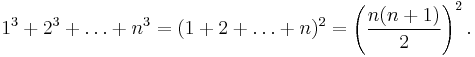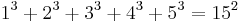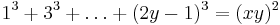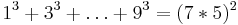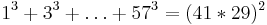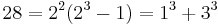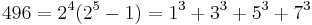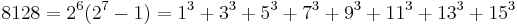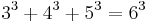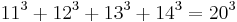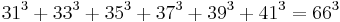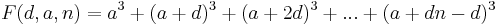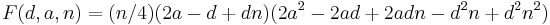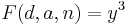Cube (algebra)

In arithmetic and algebra, the cube of a number n is its third power — the result of the number multiplying by itself three times:

n³ = n × n × n.

This is also the volume formula for a geometric cube with sides of length n, giving rise to the name. The inverse operation of finding a number whose cube is n is called extracting the cube root of n. It determines the side of the cube of a given volume. It is also n raised to the one-third power.

A cube number usually has a small 3 above it for example x³.

A perfect cube (also called a cube number, or sometimes just a cube) is a number which is the cube of an integer.

The sequence of non-negative perfect cubes starts (sequence A000578 in OEIS):

0, 1, 8, 27, 64, 125, 216, 343, 512, 729, 1000, 1331, 1728, 2197, 2744, 3375, 4096, 4913, 5832, 6859, 8000, 9261, 10648, 12167, 13824, 15625, 17576, 19683, 21952, 24389, 27000, 29791, 32768, 35937, 39304, 42875, 46656, 50653, 54872, 59319, 64000, 68921, 74088, 79507, 85184, 91125, 97336, 103823, 110592, 117649, 125000, 132651, 140608, 148877, 157464, 166375, 175616, 185193, 195112, 205379, 216000, 226981, 238328...

Geometrically speaking, a positive number m is a perfect cube if and only if one can arrange m solid unit cubes into a larger, solid cube. For example, 27 small cubes can be arranged into one larger one with the appearance of a Rubik's Cube, since 3 × 3 × 3 = 27.

The pattern between every perfect cube from negative infinity to positive infinity is as follows,

n³ = (n − 1)³ + 3(n − 1)n + 1.

n³ = (n + 1)³ − 3(n + 1)n − 1.

1 Cubes in number theory
2 History
3 Notes
4 References
5 See also
6 External links

Cubes in number theory

There is no smallest perfect cube, since negative integers are included. For example, (−4) × (−4) × (−4) = −64. For any n, (−n)³ = −(n³).

Base ten

Unlike perfect squares, perfect cubes do not have a small number of possibilities for the last two digits. Except for cubes divisible by 5, where only 25, 75 and 00 can be the last two digits, any pair of digits with the last digit odd can be a perfect cube. With even cubes, there is considerable restriction, for only 00, o2, e4, o6 and e8 can be the last two digits of a perfect cube (where o stands for any odd digit and e for any even digit). Some cube numbers are also square numbers, for example 64 is a square number (8 × 8) and a cube number (4 × 4 × 4); this happens if and only if the number is a perfect sixth power.

It is, however, easy to show that most numbers are not perfect cubes because all perfect cubes must have digital root 1, 8 or 9. Moreover, the digital root of any number's cube can be determined by the remainder the number gives when divided by 3:

If the number is divisible by 3, its cube has digital root 9;
If it has a remainder of 1 when divided by 3, its cube has digital root 1;
If it has a remainder of 2 when divided by 3, its cube has digital root 8.

Waring's problem for cubes

Main article: Waring's problem

Every positive integer can be written as the sum of nine (or fewer) positive cubes. This upper limit of nine cubes cannot be reduced because, for example, 23 cannot be written as the sum of fewer than nine positive cubes:

23 = 2³ + 2³ + 1³ + 1³ + 1³ + 1³ + 1³ + 1³ + 1³.

Fermat's last theorem for cubes

Main article: Fermat's last theorem

The equation x³ + y³ = z³ has no non-trivial (i.e. xyz ≠ 0) solutions in integers. In fact, it has none in Eisenstein integers.^[1]

Both of these statements are also true for the equation^[2] x³ + y³ = 3z³.

Sums of rational cubes

Every positive rational number is the sum of three positive rational cubes,^[3] and there are rationals that are not the sum of two rational cubes.^[4]

Sum of first n cubes

The sum of the first n cubes is the n^th triangle number squared:

$1^3%2B2^3%2B\dots%2Bn^3 = (1%2B2%2B\dots%2Bn)^2=\left(\frac{n(n%2B1)}{2}\right)^2.$

For example, the sum of the first 5 cubes is the square of the 5th triangular number,

$1^3%2B2^3%2B3^3%2B4^3%2B5^3 = 15^2 \,$

A similar result can be given for the sum of the first y odd cubes,

$1^3%2B3^3%2B\dots%2B(2y-1)^3 = (xy)^2$

but {x,y} must satisfy the negative Pell equation 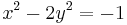$x^2-2y^2 = -1$ . For example, for y = 5 and 29, then,

$1^3%2B3^3%2B\dots%2B9^3 = (7*5)^2 \,$

$1^3%2B3^3%2B\dots%2B57^3 = (41*29)^2$

and so on. Also, every even perfect number, except the first one, is the sum of the first 2^(p−1)/2 odd cubes,

$28 = 2^2(2^3-1) = 1^3%2B3^3$

$496 = 2^4(2^5-1) = 1^3%2B3^3%2B5^3%2B7^3$

$8128 = 2^6(2^7-1) = 1^3%2B3^3%2B5^3%2B7^3%2B9^3%2B11^3%2B13^3%2B15^3$

Sum of cubes in arithmetic progression

There are examples of cubes in arithmetic progression whose sum is a cube,

$3^3%2B4^3%2B5^3 = 6^3$

$11^3%2B12^3%2B13^3%2B14^3 = 20^3$

$31^3%2B33^3%2B35^3%2B37^3%2B39^3%2B41^3 = 66^3$

with the first one also known as Plato's number. The formula F for finding the sum of an n number of cubes in arithmetic progression with common difference d and initial cube a³,

$F(d,a,n) = a^3%2B(a%2Bd)^3%2B(a%2B2d)^3%2B...%2B(a%2Bdn-d)^3$

is given by,

$F(d,a,n) = (n/4)(2a-d%2Bdn)(2a^2-2ad%2B2adn-d^2n%2Bd^2n^2)$

A parametric solution to,

$F(d,a,n) = y^3$

is known for the special case of d = 1, or consecutive cubes, but only sporadic solutions are known for integer d > 1, such as d = {2,3,5,7,11,13,37,39}, etc.^[5]

History

Determination of the Cube of large numbers was very common in many ancient civilizations. Aryabhata, the ancient Indian mathematician in his famous work Aryabhatiya explains about the mathematical meaning of cube (Aryabhatiya, 2-3), as "the continuous product of three equals as also the (rectangular) solid having 12 equal edges are called cube". Similar definitions can be seen in ancient texts such as Brahmasphuta Siddhanta (XVIII. 42) , Ganitha sara sangraha (II. 43) and Siddhanta sekhara (XIII. 4). It is interesting that in modern mathematics too, the term "Cube" stands for two mathematical meanings just like in Sanskrit , where the word Ghhana means a factor of power with the number, multiplied by itself three times and also a cubical structure.

Notes

^ Hardy & Wright, Thm. 227
^ Hardy & Wright, Thm. 232
^ Hardy & Wright, Thm. 234
^ Hardy & Wright, Thm. 233
^ >"A Collection of Algebraic Identities". http://sites.google.com/site/tpiezas/update06.

References

Hardy, G. H.; Wright, E. M. (1980). An Introduction to the Theory of Numbers (Fifth edition). Oxford: Oxford University Press. ISBN 978-0198531715

External links

A Java applet that decomposes an integer number not congruent to 4 or 5 (mod 9) into a sum of four cubes.